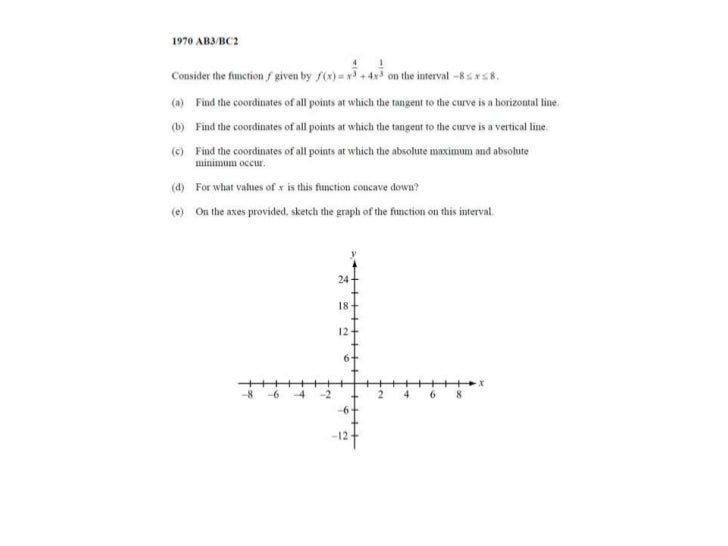
7advanced Integration & Applicationap Calculus
Advanced Integration Group, Inc. is a full service engineering firm that provides a wide variety of traditional and specialized engineering and support services. AIG is comprised of three divisions:
ADVANCED INTEGRATION GROUP ? Control and Information Systems Integration, Consulting Engineers, PLC, DCS & SCADA Programming, Panel Design & Fabrication, Construction & Facilities Management, Calibrating Instrumentation and Electrical Equipment, Electrical Contracting.
ADVANCED TECHNICAL STAFFING ? Provides temporary/contract and permanent employees and associated placement services for engineering, technical, specialized administrative and IT personnel.
ADVANCED TECHNICAL PRODUCTS ? Distributor for Scientific Technologies Inc.- Automation Products; Islip Flow Controls ? Pipeline Strainers and Valves; Ambar - Oil Separation & Filtration Products; Roediger- Biosolids Removal Sludge Thickening & Dewatering Equipment; Severn Trent ? Reverse Osmosis Water Purification Solutions; Hanna Instruments ? Meters and Controllers; and Clear-Com ? Communication Equipment
AIG is a fiscally sound corporation with experience and capabilities in all areas of the controls industry, staffing and technical product sales. Our engineering personnel have a very diverse background with experience on most major control hardware platforms in many industries. We have experience with domestic and international projects providing turnkey systems, equipment, integration work and on-site start-up and installation support. Our staffing division ATS has a wide range of qualified personnel including IT professionals, project managers, engineers, designers, and technicians along with a variety of other specialized technical and administrative personnel. We work with clients to address an entire spectrum of needs, handling everything from engineering design to implementation in order to deliver comprehensive and cost effective services. Our ATP sales and engineering staff have many years of process experince in the automation, controls, water and filtration marketplace.
AIG is able to provide services to federal, state, and local governments; private sector corporations; non-profit organizations and institutions; business and industry. We can oversee day-to-day engineering, construction and maintenance activities at client sites. In this role AIG is pleased to offer the following services: Preventive Maintenance Program Implementatoin and Execution, Construction Management, Facilities Management, Project Management, Project Scheduling, Engineering and Support Personnel Management, etc.
Advanced Integration Group, Inc. is adept at helping clients meet constantly changing governmental and business demands. Engineering Service Offered: Control Systems Project Design and Implementation, PLC, SCADA, DCS, HMI Programming, Retrofit Project Design and Implementation, Start-Up and Commissioning Services, Field Service Engineering for Instrumentation, Valves and other Industrial Equipment, Cad Design and Documentation Service, Panel Design and Fabrication Services, etc.
Advanced Integration Group, Inc. works with clients to provide innovative solutions that meet our clients? diverse business needs. Consulting service offerings include: I&C Engineers, Electrical Engineers, Mechanical Engineers, Process Engineers, Software Engineers, Programmers, Web Developers, Database Developers, SCADA Developers, Network Engineers and Administrators, Helpdesk Personnel, CAD Designers (all disciplines), Field Service Engineers, Technicians, Registered Professional Engineers, Civil and Structural Engineers, Project Managers, Project Schedulers, etc.
Advanced Integration Group, Inc. is certified as a participant in the US Small Business Administrations? (SBA) 8(A) Business Development (BD) Program and as a Small Disadvantaged Business (SDB); has an SBA approved Mentor/Prot駩 Agreement with Wellington Power, electrical contractor. Certified by the following: WBE-DGS(PA), MWBE, City of Charlotte, NC and DBE?UCP(PA).
In addition, Advanced Integration Group, Inc. is a 'certified' member of the CSIA - Control Systems Integrator's Association

The SIMATIC PCS 7 Industry Library can be used to create harmonious overall solutions for specific I&C tasks in numerous industrial sectors. It offers block symbols and faceplates in the look & feel of the SIMATIC PCS 7 Advanced Process Library. This allows the development of tailor-made industry applications with a uniform operating philosophy. Advanced Examples On Integration Set-4 in Indefinite Integration with concepts, examples and solutions. FREE Cuemath material for JEE,CBSE, ICSE for excellent results! Joget DX Available for Download. Check out Joget DX, the next generation successor to Joget Workflow for faster, simpler digital transformation. LearnDash is a popular WordPress LMS plugin packed with lots of features and has worldwide clients. To make your LearnDash LMS a complete learning environment, you can use the following plugins to make it advanced and user-friendly.
We now consider more irrational algebraic expressions. The forms that are generally encountered are listed below along with the recommended substitutions:
(a) (begin{align}int {frac{1}{{{L_1}left( x right)sqrt {{L_2}left( x right)} }}} ,dx qquad :;;;{text{Substitute}},{L_2}left( x right) = {t^2}end{align})
(b) (begin{align}int {frac{1}{{Lleft( x right)sqrt {Qleft( x right)} }}} ,dx qquad :;;;;{text{Substitute}},Lleft( x right) = frac{1}{t}end{align})
(c) (begin{align}int {frac{1}{{Qleft( x right)sqrt {Lleft( x right)} }}} ,dx qquad :;;;{text{Substitute}},Lleft( x right) = {t^2}end{align})

(d) (begin{align}int {frac{1}{{{Q_1}left( x right)sqrt {{Q_2}left( x right)} }}} ,dx qquad :;;;;{text{Substitute}},frac{{{Q_2}left( x right)}}{{{Q_1}left( x right)}} = {t^2}left( begin{gathered}{text{If the expressions}}{Q_1}left( x right){text{ and }}{Q_2}left( x right){text{are}},{text{purely quadratic}},{text{i}}{text{.e}},{text{the coefficients}}{text{of }}x,{text{are both are zero}},{text{the}}{text{substitution}};x = frac{1}{t}{text{would also do }}end{gathered} right)end{align})
Some examples will make the use of these substitutions more clear
Example – 56
Evaluate the following integrals:
(a) (begin{align}int {frac{1}{{left( {x - 1} right)sqrt {x + 2} }}} ,dx end{align})
(b) (begin{align}int {frac{x}{{left( {x - 3} right)sqrt {x + 1} }}} ,dxend{align})
(c) (begin{align}int {frac{1}{{left( {x + 1} right)sqrt {{x^2} + x + 1} }}} ,dx end{align})
(d) (begin{align}int {frac{x}{{left( {{x^2} + 2x + 2} right)sqrt {x + 1} }}} ,dx end{align})

Solution: Refer to the substitutions mentioned in the preceeding discussion:
(a) Substitute
[begin{align}& qquad x+2={{t}^{2}} & Rightarrow quad dx=2,tdt & Rightarrowquad I=int{frac{1}{left( x-1 right)sqrt{x+2}}},dx &qquadquad =int{frac{1}{left( {{t}^{2}}-3 right)cdot t}}cdot ,2,tdt &qquadquad =2int{frac{1}{{{t}^{2}}-3}},dt &qquadquad =frac{1}{sqrt{3}}ln left| frac{t-sqrt{3}}{t+sqrt{3}} right|+C &qquadquad =frac{1}{sqrt{3}}ln left| frac{sqrt{x+2}-sqrt{3}}{sqrt{x+2}+sqrt{3}} right|+C end{align}]
(b) Substitute
[begin{align} &qquad x + 1 = {t^2} & Rightarrowquad dx=2tdt & Rightarrow quad I=int{frac{x}{left( x-3 right)sqrt{x+1}}}dx & qquadquad =int{frac{left( {{t}^{2}}-1 right)}{left( {{t}^{2}}-4 right)cdot t}}2tdt & qquadquad=2int{frac{{{t}^{2}}-1}{{{t}^{2}}-4}}dt & qquadquad=2int{left{ 1+frac{3}{{{t}^{2}}-4} right}}dt & qquadquad=2t+frac{3}{2}ln left| frac{t-2}{t+2} right|+C & qquadquad =2sqrt{x+1}+frac{3}{2}ln left| frac{sqrt{x+1}-2}{sqrt{x+1}+2} right|+C end{align}]
(c) Substitute
[begin{align}&qquad x + 1 = frac{1}{t}& Rightarrow quad dx=-frac{1}{{{t}^{2}}}dt & Rightarrow quad I=int{frac{1}{left( x+1 right)sqrt{{{x}^{2}}+x+1}}}dx &qquadquad=int{frac{t}{sqrt{{{left( frac{1}{t}-1 right)}^{2}}+left( frac{1}{t}-1 right)+1}}}cdot left( -frac{1}{{{t}^{2}}} right)dt &qquadquad=-int{frac{1}{sqrt{{{t}^{2}}-t+1}}}dt &qquadquad=-int{frac{1}{sqrt{{{left( t-frac{1}{2} right)}^{2}}+frac{3}{4}}}}dt &qquadquad=-ln left| left( t-frac{1}{2} right)+sqrt{{{t}^{2}}-t+1} right|+C & qquadquad =-ln left| frac{1}{x+1}-frac{1}{2}+frac{sqrt{{{x}^{2}}+x+1}}{x+1} right|+C end{align}]
(d) Substitute
[begin{align} &qquadquad x+1={{t}^{2}} &Rightarrow quad dx=2tdt &Rightarrowquad I=int{frac{x}{left( {{x}^{2}}+2x+2 right)sqrt{x+1}}}dx &qquadquad =int{frac{{{t}^{2}}-1}{left( {{t}^{4}}+1 right)cdot t}}cdot 2tdt & qquadquad=2int{frac{{{t}^{2}}-1}{{{t}^{4}}+1}}dt end{align}]

7 Advanced Integration & Application Ap Calculus Frq
We’ve already seen how to evaluate integrals of this type
