1. 3.4 Derivative Of E^f(x) And Ln (f(x))ap Calculus 2nd Edition
2. 3.4 Derivative Of E^f(x) And Ln (f(x))ap Calculus 14th Edition
3. 3.4 Derivative Of E^f(x) And Ln (f(x))ap Calculus Calculator
4. 3.4 Derivative Of E^f(x) And Ln (f(x))ap Calculus Solver

The Derivative of the Natural Logarithm

Derivation of the Derivative

Our next task is to determine what is the derivative of the natural logarithm. We begin with the inverse definition. If

Compute the derivatives of the following functions (a) f(x) = x 2 + 1 x − 3.03x + 4 (b) g(x) = ln(x) (c) h(x) = x 2 (x 3 + 4)5 (d) f(x) = x2 x (e) f(x) = e 4x (f) f(x) = e x 1 + x 2. Let f(x) = x 3 − 3x 2 + 0.5x − 2. (a) Compute the average rate of change of f(x) from x = −1 to x = 1. (b) Draw an accurate graph of f(x) with x between. This calculator evaluates derivatives using analytical differentiation. It will also find local minimum and maximum, of the given function.The calculator will try to simplify result as much as possible.

y = ln x

then

e^y = x

Now implicitly take the derivative of both sides with respect to x remembering to multiply by dy/dx on the left hand side since it is given in terms of y not x.

e^y dy/dx = 1

From the inverse definition, we can substitute x in for e^y to get

x dy/dx = 1

Finally, divide by x to get

dy/dx = 1/x

We have proven the following theorem

Examples

Find the derivative of

f(x) = ln(3x - 4)

Solution

We use the chain rule. We have

(3x - 4)' = 3

and

(ln u)' = 1/u

Putting this together gives

f '(x) = (3)(1/u)

3
=
3x - 4

Example

find the derivative of

f(x) = ln[(1 + x)(1 + x²)²(1 + x³)³ ]

Solution

The last thing that we want to do is to use the product rule and chain rule multiple times. Instead, we first simplify with properties of the natural logarithm. We have

ln[(1 + x)(1 + x²)²(1 + x³)³ ] = ln(1 + x) + ln(1 + x²)² + ln(1 + x³)³

= ln(1 + x) + 2 ln(1 + x²) + 3 ln(1 + x³)

Now the derivative is not so daunting. We have use the chain rule to get

1 4x 9x²
f '(x) = + +
1 + x 1 + x² 1 + x³

Exponentials and With Other Bases

Examples
Find the derivative of

f (x) = 2^x

Solution

We write
2^x = e^{x ln 2}

Now use the chain rule
f '(x) = (e^{x ln 2})(ln 2) = 2^x ln 2

Logs With Other Bases

We define logarithms with other bases by the change of base formula.

Remark: The nice part of this formula is that the denominator is a constant. We do not have to use the quotient rule to find a derivative

Examples
Find the derivative of the following functions

3.4 Derivative Of E^f(x) And Ln (f(x))ap Calculus 2nd Edition

1. f(x) = log₄ x
   

2. f(x) = log (3x + 4)
   

3. f(x) = x log(2x)
   

Solution

1. We use the formula
   ln x
   f(x) =
   ln 4
   
   so that
   1
   f '(x) =
   x ln 4
   
   

2. We again use the formula
   
   ln(3x + 4)
   f(x) =
   ln 10
   
   
   now use the chain rule to get
   3
   f '(x) =
   (3x + 4) ln 10
   
   

3. Use the product rule to get
   f '(x) = log(2x) + x(log(2x))'
   
   Now use the formula to get
   ln(2x)
   log (2x) =
   ln 10
   
   The chain rule gives
   2 1
   f '(x) = log(2x) + x = log(2x) +
   (2x) ln 10 ln 10
   
   

The Derivative Calculator lets you calculate derivatives of functions online — for free!

Our calculator allows you to check your solutions to calculus exercises. It helps you practice by showing you the full working (step by step differentiation).

The Derivative Calculator supports computing first, second, …, fifth derivatives as well as differentiating functions with many variables (partial derivatives), implicit differentiation and calculating roots/zeros. You can also check your answers! Interactive graphs/plots help visualize and better understand the functions.

For more about how to use the Derivative Calculator, go to 'Help' or take a look at the examples.

And now: Happy differentiating!

Enter the function you want to differentiate into the Derivative Calculator. Skip the 'f(x) =' part! The Derivative Calculator will show you a graphical version of your input while you type. Make sure that it shows exactly what you want. Use parentheses, if necessary, e. g. 'a/(b+c)'.

In 'Examples', you can see which functions are supported by the Derivative Calculator and how to use them.

When you're done entering your function, click 'Go!', and the Derivative Calculator will show the result below.

In 'Options' you can set the differentiation variable and the order (first, second, … derivative). You can also choose whether to show the steps and enable expression simplification.

Clicking an example enters it into the Derivative Calculator. Moving the mouse over it shows the text.

Configure the Derivative Calculator:

The practice problem generator allows you to generate as many random exercises as you want.

You find some configuration options and a proposed problem below. You can accept it (then it's input into the calculator) or generate a new one.

Calculate the Derivative of …Enter your own Answer:

3.4 Derivative Of E^f(x) And Ln (f(x))ap Calculus 14th Edition

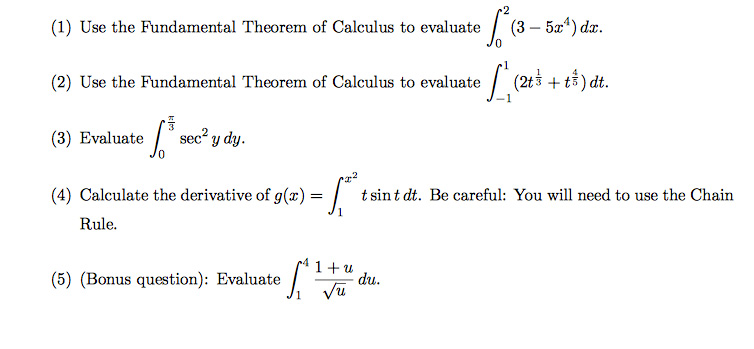
This will be calculated:

Not what you mean? Use parentheses! Set differentiation variable and order in 'Options'.

Recommend this Website

If you like this website, then please support it by giving it a Like. Thank you!

Book Recommendation

Calculus for Dummies (2nd Edition)

An extremely well-written book for students taking Calculus for the first time as well as those who need a refresher. This book makes you realize that Calculus isn't that tough after all. → to the book

Paid link. As an Amazon Associate I earn from qualifying purchases.

Result

How the Derivative Calculator Works

For those with a technical background, the following section explains how the Derivative Calculator works.

First, a parser analyzes the mathematical function. It transforms it into a form that is better understandable by a computer, namely a tree (see figure below). In doing this, the Derivative Calculator has to respect the order of operations. A specialty in mathematical expressions is that the multiplication sign can be left out sometimes, for example we write '5x' instead of '5*x'. The Derivative Calculator has to detect these cases and insert the multiplication sign.

The parser is implemented in JavaScript, based on the Shunting-yard algorithm, and can run directly in the browser. This allows for quick feedback while typing by transforming the tree into LaTeX code. MathJax takes care of displaying it in the browser.

When the 'Go!' button is clicked, the Derivative Calculator sends the mathematical function and the settings (differentiation variable and order) to the server, where it is analyzed again. This time, the function gets transformed into a form that can be understood by the computer algebra systemMaxima.

Maxima takes care of actually computing the derivative of the mathematical function. Like any computer algebra system, it applies a number of rules to simplify the function and calculate the derivatives according to the commonly known differentiation rules. Maxima's output is transformed to LaTeX again and is then presented to the user.

Displaying the steps of calculation is a bit more involved, because the Derivative Calculator can't completely depend on Maxima for this task. Instead, the derivatives have to be calculated manually step by step. The rules of differentiation (product rule, quotient rule, chain rule, …) have been implemented in JavaScript code. There is also a table of derivative functions for the trigonometric functions and the square root, logarithm and exponential function. In each calculation step, one differentiation operation is carried out or rewritten. For example, constant factors are pulled out of differentiation operations and sums are split up (sum rule). This, and general simplifications, is done by Maxima. For each calculated derivative, the LaTeX representations of the resulting mathematical expressions are tagged in the HTML code so that highlighting is possible.

3.4 Derivative Of E^f(x) And Ln (f(x))ap Calculus Calculator

The 'Check answer' feature has to solve the difficult task of determining whether two mathematical expressions are equivalent. Their difference is computed and simplified as far as possible using Maxima. For example, this involves writing trigonometric/hyperbolic functions in their exponential forms. If it can be shown that the difference simplifies to zero, the task is solved. Otherwise, a probabilistic algorithm is applied that evaluates and compares both functions at randomly chosen places.

3.4 Derivative Of E^f(x) And Ln (f(x))ap Calculus Solver

The interactive function graphs are computed in the browser and displayed within a canvas element (HTML5). For each function to be graphed, the calculator creates a JavaScript function, which is then evaluated in small steps in order to draw the graph. While graphing, singularities (e. g. poles) are detected and treated specially. The gesture control is implemented using Hammer.js.

If you have any questions or ideas for improvements to the Derivative Calculator, don't hesitate to write me an e-mail.